The Peltier effect occurs when current flows between two dissimilar conductors or semiconductors. When this occurs, the charge carriers flowing through the material will transfer heat from one side to the other, allowing this effect to be used to create a heat pump.[1] The power (heat per unit time) generated by the effect can be calculated by the following equation:
where and are the Peltier coefficients of the two materials and I is the electrical current.[1]Thus it can be seen that the power of the device is dependent on the difference between the Peltier coefficients of the two materials.
- The Seebeck effect
The Seebeck effect is a variation of the Peltier effect and is essentially the same process carried out in reverse. When two dissimilar conductors or semiconductors are connected and are at different temperatures, a voltage between them will be created.[1]The Seebeck coefficient can be calculated using the following equation:
where SA and SB are the Seebeck coefficients of the two materials, is the voltage difference, and is the temperature difference.[2] The relationship between the Peltier coefficient and the Seebeck coefficient (which is an intrinsic material property) can is given by the following relationship:
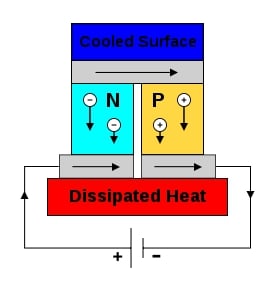
;[1]Thermoelectric cooling/heating As shown on the right, a typical thermoelectric cooling unit consists of a series of P and N type semiconductors wired electrically in series and thermally in parallel (direction of heat flow is determined by direction of current flow and whether it is P-type or N-type). Since metals often have a very low (absolute value) Seebeck coefficient, the conductive metal (typically copper) used to connect the semiconductors together also acts as the material that is 'different' (in terms of Seebeck coefficient) from the semiconductor itself.[1]
Description of Material[edit | edit source]
- For our device, we will be using two types of semiconductors, Bi2Te3 (Bismuth Telluride, N-type) and Sb2Te3 (Antimony Telluride, P-type). We also be using a conductor (97% Indium 3% Silver alloy), a Colloidal Ink (Polyethylenimine, C2H5N) and an insulator (Silicone rubber). The two semiconductors were chosen for having high Seebeck coefficients with opposite signs (Bi2Te3: -230 and Sb2Te3: 185 )[3] as well as having high electrical conductivity and low thermal conductivity. As described below, it has also been shown that these materials can be successfully 3-D printed. The Indium Silver alloy, like most metals, has a relatively low (absolute value) Seebeck coefficient, thus serving as a good choice for an electrical contact. It also has high electrical and thermal conductivity. Silicone is both electrically insulating and thermally conductive making it an ideal choice to form the outer shell surrounding the entire device.
| Chemical Name/MSDS | Cost (unit) | Supplier |
|---|---|---|
| Indium Silver Alloy | $201/30x30 mm foil | Goodfellow Corporation |
| Bismuth Telluride | $52.00/5 gallons | Sigma Aldrich |
| Antimony Telluride | $22.60/1 gallon | Sigma Aldrich |
| Silicone Rubber | $6.58/3 oz. | GC electronics |
| Polyethylenimine Ink | $46.10/10 ML | Sigma Aldrich |
| Material | Electrical Conductivity | Thermal Conductivity | Seebeck coefficient | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Indium Silver Alloy | 1.3×10^7Ω-1·m-1[4] | ~0, metal alloy | |||||||||
| Bismuth Telluride | 1.1×10^5 Ω-1·m-1 | 1.20 W/m·K | - | Antimony Telluride | 2.7*10^5Ω-1·m-1 | N/A | - | Silicone Rubber | 0.1Ω-1·m-1 | 1.2 W/m·K | N/A |
3-D printing of Material[edit | edit source]
For low temperature printing of the semiconductors used in our device we have found two potential methods. The first is directed colloidal assembly in which a charged, anionic polyelectrolyte is used to coat microstructures of the desired semiconductor that is then turned into a gel by varying the pH of the solution and when printed sets nearly immediately. Careful selection of the polyelectrolyte is needed to achieve the desired gel formation and semiconductor density in the ink.[5] The second method is semi-solid metal casting (SSM) that takes advantage of the thixotropy fluid property which has been shown to be applicable to non-ferrus metals such as copper, aluminum, and bismuth. For SSM the alloy must be brought to a temperature between its solidus and liquidus temperatures, between 5 °C and 10 °C for aluminum alloys for example, and a shearing force must be applied to get the desired structure for printing, which can be provided by an auger or screw. Determination of the correct temperature for SSM for the semiconductors of interest and use of an printer setup to handle clay like material are needed for the use of this ink formulation.
Indium Silver alloy has a melting point of 143C and can thus be easily melted and extruded through a RepRap printer.[6] Silicone rubber can also be extruded using methods similar to those used in commercial silicone sealant applications and does not need to be melted.
Inconel alloy is a family of nickel-chromium super alloys and a trademark of Special Metals Corporation. It is typically used in high temperature applications.[7] 600 (UNS N06600) is often used for applications which require resistance to corrosion and heat.
Dia Workflow[edit | edit source]
Purification[edit | edit source]
Bismuth telluride, Bi2Te3, and antimony telluride, Sb2Te3, need to have high purity to ensure optimal carrier concentration, electrical conductivity, and thermal conductivity.[8] Typically pure, approximately 99.99%, bismuth, antimony, and tellurium are used as a starting point to achieve a pure final product. Pure bismuth or antimony, and tellurium are alloyed by mechanical alloying in a ball mill to produce a powder which can then be extruded to form an ingot or pellet if desired. This method produces high purity Bi2Te3 or Sb2Te3 in bulk with growth orientation in the extruded direction and strong mechanical properties.[9] Alternatively, metal salts of bismuth and tellurium can be used to create Bi2Te3 through reaction with thiols, which creates a high purity alloy with a desirable nano-structure compared to ball milling.[10] Sb2Te3 can most likely be purified via chemical reactions in a similar manner, though we did not find any literature data on the subject. Bismuth is a high-density, silvery, pink-tinged metal. Bismuth metal is brittle and so it is usually mixed with other metals to make it useful. Its alloys with tin or cadmium have low melting points and are used in fire detectors and extinguishers, electric fuses and solders.
Characterization[edit | edit source]
- Direct measurement of Seebeck Coefficient
Directly measuring the Seebeck Coefficient of the semiconductor in-situ is rather difficult, but not impossible. Using two copper blocks in contact with the semiconductor portion of the device, a differential thermocouple can be created. Then, the voltage between the two blocks can be measured as the temperature of one of the blocks is precisely varied. Connecting the two blocks at a separate location with a material with a known Seebeck coefficient and measuring the voltage difference at that point will allow accurate measurement of the temperature difference between the blocks. Then finally the Seebeck Coefficient of the printed semiconductor can be calculated.[2]Due to the sensitive nature of the physical phenomena, the entire apparatus will need to be in an isolated environment and the printer itself will need to be completely shut down and the heated components allowed to cool. See reference for further reading.
- Alternative method of in-situ characterization
A cruder and much simpler method of testing the properties of the device would be to simply turn it on. The electrical circuit itself is very simple and only requires a current flow, thus turning the device on would simply mean connecting it to a power supply. Once turned on, the increase/decrease in temperature around the device can be measured which, in terms of functionality, is sufficient.
Applications[edit | edit source]
- Thermoses
- Laser cooling
- Electronics refrigeration
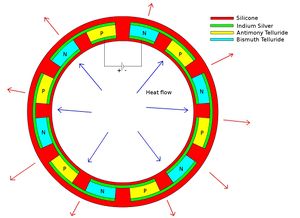
- Advantage of 3-D printing
- Conventionally, semiconductor pellets used in Peltier cooling devices were machined to be rectangular blocks and arranged into a flat grid. However, with 3-D printing, each individual component can be made to whatever shape and size is necessary, thus allowing the entire cooling unit to take shapes and forms previously impossible (or very difficult) to make. Each unit can be custom shaped to perfectly fit the application it is being used for allowing for new possibilities in design for these applications. 3-D printing also removes the need to assemble this fairly complex device piece by piece, instead allowing it to efficiently printed using a single machine.
OpenSCAD[edit | edit source]
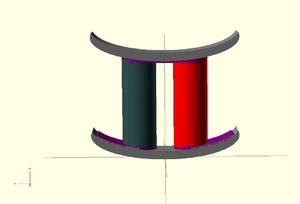
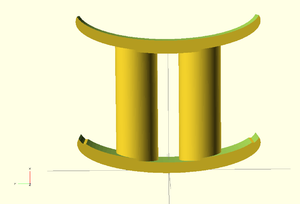
− //Curved Peltier Cooler Unit
//semiconductors
// + y-direction {color("DarkSlateGrey") union(){ //shaft translate([0.73,0.75,0]) rotate([0,90,0]) cylinder(h=2,r=0.5,$fn=100);
//bottom intersection() { translate([1,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); translate([0,0.75,0]) rotate([0,90,0]) cylinder(h=1,r=0.5,$fn=100); } //top difference() { translate([2.5,0.75,0]) rotate([0,90,0]) cylinder(h=1,r=0.5,$fn=100); translate([3.5,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } } }
// - y-direction {color("Red") union(){ //shaft translate([0.73,-0.75,0]) rotate([0,90,0]) cylinder(h=2,r=0.5,$fn=100); //bottom intersection() { translate([1,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); translate([0,-0.75,0]) rotate([0,90,0]) cylinder(h=1,r=0.5,$fn=100); } //top difference() { translate([2.5,-0.75,0]) rotate([0,90,0]) cylinder(h=1,r=0.5,$fn=100); translate([3.5,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } } }
//electrical conatacts
//lower {color("Purple") intersection(){ difference(){ translate([0.95,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); translate([1,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } translate([-0.12,-0.75,0]) rotate([0,90,0]) cylinder(h=0.5,r=0.5,$fn=100); } }
{color("Purple") intersection(){ difference(){ translate([0.95,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); translate([1,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } translate([-0.12,0.75,0]) rotate([0,90,0]) cylinder(h=0.5,r=0.5,$fn=100); } }
//extensions to adjacent cells
{color("Purple") intersection(){ difference(){ translate([0.95,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); translate([1,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } mirror([0,1,0]) translate([0.2,0.75,-0.5]) cube([0.5,2,1]); } }
{color("Purple") intersection(){ difference(){ translate([0.95,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); translate([1,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } translate([0.2,0.75,-0.5]) cube([0.5,2,1]); } }
//upper
union(){ //ends caps for semiconductors {color("Purple") difference(){ intersection(){ translate([2.78,-0.75,0]) rotate([0,90,0]) cylinder(h=0.5,r=0.5,$fn=100); translate([3.5,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } translate([3.55,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } }
{color("Purple") difference(){ intersection(){ translate([2.78,0.75,0]) rotate([0,90,0]) cylinder(h=0.5,r=0.5,$fn=100); translate([3.5,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } translate([3.55,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } }
//span between end caps {color("Purple") difference(){ intersection(){ translate([2.78,-0.75,-0.5]) cube([0.5,1.5,1]); translate([3.5,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } translate([3.55,0,-0.5]) resize([1.4,4.3,0]) cylinder(h=1,r=1,$fn=100); } } }
//electrically insulating thermal conductor end caps
//top {color("Grey") difference(){ translate([3.55,0,-0.5])resize([1.4,4.3,0])cylinder(h=1,r=1,$fn=100); translate([3.8,0,-0.5])resize([1.4,4.3,0])cylinder(h=1,r=1,$fn=100); } }
//bottom {color("Grey",1) difference(){ translate([0.7,0,-0.5])resize([1.4,4.3,0])cylinder(h=1,r=1,$fn=100); translate([0.95,0,-0.5])resize([1.4,4.3,0])cylinder(h=1,r=1,$fn=100); } }
STL[edit | edit source]
STL of the Basic Peltier Cell from OpenSCAD as shown above.
File:Curved Semiconductor Cell.stl
References[edit | edit source]
- ↑ 1.0 1.1 1.2 1.3 1.4 "Thermoelectric effect" http://en.wikipedia.org/wiki/Thermoelectric_effect
- ↑ 2.0 2.1 Auparay, Novela "Room Temperature Seebeck Coefficient Measurement of Metals and Semiconductors" Oregon State University http://web.archive.org/web/20131205131520/http://www.physics.oregonstate.edu/%7Etate/TateLabWiki/lib/exe/fetch.php?media=theses:auparay_bs_2013.pdf
- ↑ 3.0 3.1 3.2 Lasance, Clemens J.M. "The Seebeck Effect" Electronics Cooling http://www.electronics-cooling.com/2006/11/the-seebeck-coefficient/
- ↑ 4.0 4.1 Bolotoff, Paul V. "Solder Alloys: Physical and Mechanical Properties" http://alasir.com/reference/solder_alloys/
- ↑ James E. Smay, Gregory M. Gratson, Robert F. Shepherd, Joseph Cesarano <roman>3</roman>, and Jennifer A. Lewis Directed Colloidal Assembly of 3D Periodic Structures
- ↑ "Fusible Alloy" http://en.wikipedia.org/wiki/Fusible_alloy
- ↑ "Inconel alloy" http://www.samaterials.com/3d-printing-powder/1323-spherical-inconel-600-uns-n06600-powder-3d-printing-additive.html
- ↑ Sigma Aldrich Thermoelectrics
- ↑ N. Gothard, G. Wilks, T. M. Tritt, J. E. Spowart Process Route
- ↑ SYNTHESIS AND CHARACTERIZATION OF SEMICONDUCTOR NANOMATERIALS FOR THERMOELECTRIC APPLICATIONS A Dissertation in Chemistry by Matthew R. Dirmyer PDF







